Series in which terms alternate between positive and negative
In the infinite series notes you saw the series expansions of the sine and cosine functions. Here they are again along with the summation notation. Remember that there's nothing especially complicated about Σ notation; it's just a way of capturing what's going on in the series without having to write all or several of its terms.
$$ \begin{align} \text{sin}(x) &= \frac{x^1}{1!} - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \frac{x^9}{9!} - \dots = \sum_{n = 0}^\infty \frac{(-1)^n x^{2n + 1}}{(2n + 1)!} \\[5pt] \text{cos}(x) &= \frac{x^0}{0!} - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + \frac{x^8}{8!} - \dots = \sum_{n = 0}^\infty \frac{(-1)^n x^{2n}}{(2n)!} \end{align}$$
These are called alternating series because of the alternation in the sign of each term.
To describe such an alternation in summation notation we employ the properties of powers of negative numbers:
- $(-1)^1 = -1$
- $(-1)^2 = 1$
- $(-1)^3 = -1,$ and so on.
It's also possible to produce an alternation of sign using the trigonometric functions $\text{sin}(x)$ and $\text{cos}(x)$, like this:
- $\text{cos}(n\pi) = 1$ for even n and -1 for odd $n$
- $\text{sin}\left(\frac{n\pi}{2}\right) = 1$ for odd $n$ and -1 for even $n$
An example
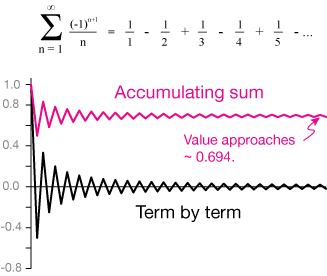
The alternating harmonic series
Here is an example of an alternating series, the so-called alternating harmonic series. This is just the harmonic series with alternating signs of the terms. The terms alternate on either side of zero as they decrease to zero (blue graph).
The accumulating sum (red graph) converges to a limit of approximately 0.69, but oscillates about that line. It's non-trivial to determine the actual sum of this series, but it does converge.
The alternating series test
The alternating series test, proved below the next box, is very simple.
It says that if, as $n \rightarrow \infty$, the terms of an alternating series decrease to zero, then the series converges.
Remember, that is NOT necessarily true for non-alternating series. For a non-alternating series, it is not enough that the size of the term diminishes; that series still may not converge.
We can think of many non-alternating, divergent series with decreasing terms, like the harmonic series:
$$\sum_{n = 1}^\infty \frac{1}{n} = 1 + \frac{1}{2} + \frac{1}{3} + \frac{1}{4} + \:\dots $$
In a way, the alternating series test (AST) makes life a lot easier. It's enough that the absolute value of the terms decrease to zero for an alternating series to converge — pretty simple.
The alternating series test
If the terms of an alternating series, $\sum_{n = 1}^\infty \,(-1)^n a_n$
(1) are decreasing, and (2) if the limit of the size of the terms, as $n \rightarrow \infty$ is zero:
then the series converges.
Proof of the alternating series test
First, take a look at the alternating series on a number line. We'll let the terms of the series be $a_1, a_2, a_3, \dots$ and so on, and the partial sums will be $s_1 = a_1, \; s_2 = a_1 + a_2, \; s_3 = a_1 + a_2 + a_3,\; \dots$ and so on.

Let's look first at the even sums. The first one (first green arrow in the diagram) is
$$s_2 = a_1 - a_2 \gt 0 \; \leftarrow \; \text{ because } a_1 \gt a_2$$
The second and third are
$$ \begin{align} s_4 &= s_2 + (a_3 - a_4) \ge s_2 \; \leftarrow \; \text{ because } a_3 \gt a_4 \\[5pt] s_6 &= s_4 + (a_5 - a_6) \ge s_4 \; \leftarrow \; \text{ because } a_5 \gt a_6 \end{align}$$
... and the pattern emerges. In the notation below, $2n$ is always an even number, $2n-2$ is the next smaller even number and $2n-1$ is the next smaller odd number:
$$s_{2n} = s_{2n - 2} + (a_{2n - 1} - a_{2n}) \ge s_{2n - 2}$$
Now it's clear that these sums continue to grow, so that
$$0 \le s_2 \le s_4 \le s_6 \le \dots \le s_{2n} \le \dots$$
By regrouping terms with some parenthesis, we can also write $s_{2n}$ as
$$s_{2n} = a_1 - (a_2 - a_1) - (a_4 - a_3) - \dots - (a_{2n - 2} - a_{2n - 1}) - a_{2n}$$
Now because our terms are decreasing, every difference in parenthesis is positive. The sequence of terms $\{s_n\}$ is positive, increasing and bounded from above by the sum of the series, $s$ (see diagram). It therefore has a limit,
$$\lim_{n\to\infty} s_{2n} = s$$
Now because $s_{2n + 1} = s_{2n} + a+{2n + 1},$ we can find the limit of the odd partial sums:
$$\lim_{n\to\infty} s_{2n + 1} = \lim_{n\to\infty} (s_{2n} + a_{2n + 1})$$
What we've shown is that the limits of the even and odd partial sums is the same. This is called "squeezing." If the limits from above and below are $s$, the sum of the series, then the series must converge to $s$.
The alternating series test makes determining the convergence of alternating series much easier than that of a non-alternating series. If the series alternates in sign, and if its terms decrease toward zero, the series converges.
Refining the idea of convergence
The difference in convergence between the alternating harmonic series,
$$\sum_{n = 1}^\infty \, (-1)^n \frac{1}{n}$$
which converges, and the harmonic series
$$\sum_{n = 1}^\infty \, \frac{1}{n}$$
which doesn't, hints at a subtle differentiation in how series converge. In fact, the alternating harmonic series is called "conditionally convergent," the condition being that its terms alternate sign.
On the other hand, a series like the convergent p-series,
$$\sum_{n = 1}^\infty \, \frac{1}{n^2}$$
and its alternating series counterpart,
$$\sum_{n = 1}^\infty \, (-1)^n \frac{1}{n^2}$$
both converge, and the alternating version is said to be "absolutely convergent." Here are the rules for absolute and conditional convergence:
Absolute vs. conditional convergence
Test for absolute convergence

Test for conditional convergence

Divergence — the series diverges by any one of the tests for convergence, or the divergence test.
Example 1
Does the series $\sum_{n = 0}^\infty \, (-1)^{n + 1} \frac{2n + 3}{3n + 4}$ converge?
$$\lim_{n\to\infty} \, \frac{2n + 3}{3n + 4} = \frac{2}{3} \neq 0$$
The term does not tend toward zero, so this series does not converge. We could also say that it diverges by the divergence test. Either way, it diverges.
Example 2
Does the series $\sum_{n = 0}^\infty \, \frac{(-2)^n}{3^n}$ converge?
$$\sum_{n = 0}^\infty \, (-1)^n \left( \frac{2}{3} \right)^n$$
Now we need to find whether the limit of the term without the alternation is zero:
$$\lim_{n\to\infty} \, \left( \frac{2}{3} \right)^n = 0$$
$2/3 \lt 1$, so the limit is zero, and this alternating series converges by the AST.
Practice problems
Decide whether the following series converge.
-
$$\sum_{n = 1}^\infty \, \frac{(-1)^n}{4 + 3n}$$
Solution
$$\sum_{n = 1}^\infty \, \frac{(-1)^n}{4 + 3n}$$
1. The terms approach zero as n → ∞
$$\lim_{n\to\infty} \,\frac{1}{4 + 3n} = 0$$
2. The terms decrease:
$$ \begin{align} \frac{1}{4 + 3(n + 1)} &\lt \frac{1}{4 + 3n} \\[5pt] &\longrightarrow 4 + 3n \lt 4 + 3n + 3 \\[5pt] &\longrightarrow 0 \lt 3 \end{align}$$
3. The series is alternating, so it converges.
-
$$\sum_{n = 1}^\infty \, \frac{(-1)^n}{n^3 + 2n + 2}$$
Solution
$$\sum_{n = 1}^\infty \, \frac{(-1)^n}{n^3 + 2n + 2}$$
1. The terms approach zero as n → ∞
$$\lim_{n\to\infty} \, \frac{1}{n^3 + 2n + 2} = 0$$
2. The terms decrease:
$$ \begin{align} \frac{1}{(n + 1)^3 + 2(n + 1) + 2} &\lt \frac{1}{n^3 + 2n + 2} \\[5pt] (n + 1)^3 + 2(n + 1) + 2 &\gt n^3 + 2n + 2 \end{align}$$
3. The series is alternating, so it converges.
-
$$\sum_{n = 1}^\infty \, \frac{n \cdot \text{cos}(n\pi)}{n^2 + 1}$$
Solution
$$\sum_{n = 1}^\infty \, \frac{n \cdot \text{cos}(n\pi)}{n^2 + 1}$$
The term cos(nπ) makes this an alternating series: \text{cos}(0\pi) = 0, \; \text{cos}(1\pi) = -1, \text{cos}(2\pi) = 1$, and so on.
1. The terms approach zero as $n \rightarrow \infty$
$$\lim_{n\to\infty} \, \frac{n}{n^2 + 1} = 0$$
2. The terms decrease:
$$ \begin{align} \frac{n + 1}{(n + 1)^2 + 1} &\lt \frac{n}{n + 1} \\[5pt] (n + 1)(n^2 + 1) &\lt n(n + 1)^2 + n \\[5pt] n^3 + n^2 + n + 1 &\lt n(n^2 + 2n + 1) + n \\[5pt] n^2 + n + 1 &\lt 2n^2 + 2n \\ 1 &\lt n^2 + n \end{align}$$
3. The series is alternating, so it converges.
-
$$\sum_{n = 1}^\infty \, \frac{(-1)^n n^2}{n^2 + 1}$$
Solution
$$\sum_{n = 1}^\infty \, \frac{(-1)^n n^2}{n^2 + 1}$$
1. The terms approach one as n → ∞
$$\lim_{n\to\infty} \, \frac{n^2}{n^2 + 1} = 1$$
So this series fails the AST because its terms don't decrease to zero in the limit as n → ∞

![]()
xaktly.com by Dr. Jeff Cruzan is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 3.0 Unported License. © 2016-2025, Jeff Cruzan. All text and images on this website not specifically attributed to another source were created by me and I reserve all rights as to their use. Any opinions expressed on this website are entirely mine, and do not necessarily reflect the views of any of my employers. Please feel free to send any questions or comments to jeff.cruzan@verizon.net.